supported by the peter c. cornell trust
Written By Julia Bozer
Cultural Program Educator
Invented in China centuries ago, tangrams are one of the oldest types of puzzle. According to some historians, they traveled around the world on trade ships in the 19th century, quickly becoming a popular parlor game in Europe and the Americas. The Chinese term for tangrams translates loosely to “the seven boards of skill.” A complete tangram set always contains seven shapes that a player must rearrange to form larger shapes and figures.With just some paper, scissors, and markers, you can make your own tangram set at home and use it to work out different configurations. This is a great game for practicing spatial reasoning, creative thinking, problem solving, and fine motor skills, while learning some core geometric concepts!
Materials
Paper
Scissors
Markers or crayons
Template: Downloadable here
Length:
Set up: 10-15 minutes
Play Time: Unlimited!
Academic Subject(s):
Social Studies, Math This lesson supports your student’s curriculum:
MATH: K.G.A.1, K.G.A.2, 1.G.A.1, 3.G.A.1, 3.G.A.2SOC: 3.4b, 3.5a
Directions:
Step 1: Print out the blank tangram set, downloadable here, or trace all of its lines onto a sheet of paper.
Step 2: Use markers or crayons to color in the shapes. Ideally, each shape should be a different color.
Step 3: Cut out each shape, following the black lines. There should be seven shapes in total. Can you name them all?
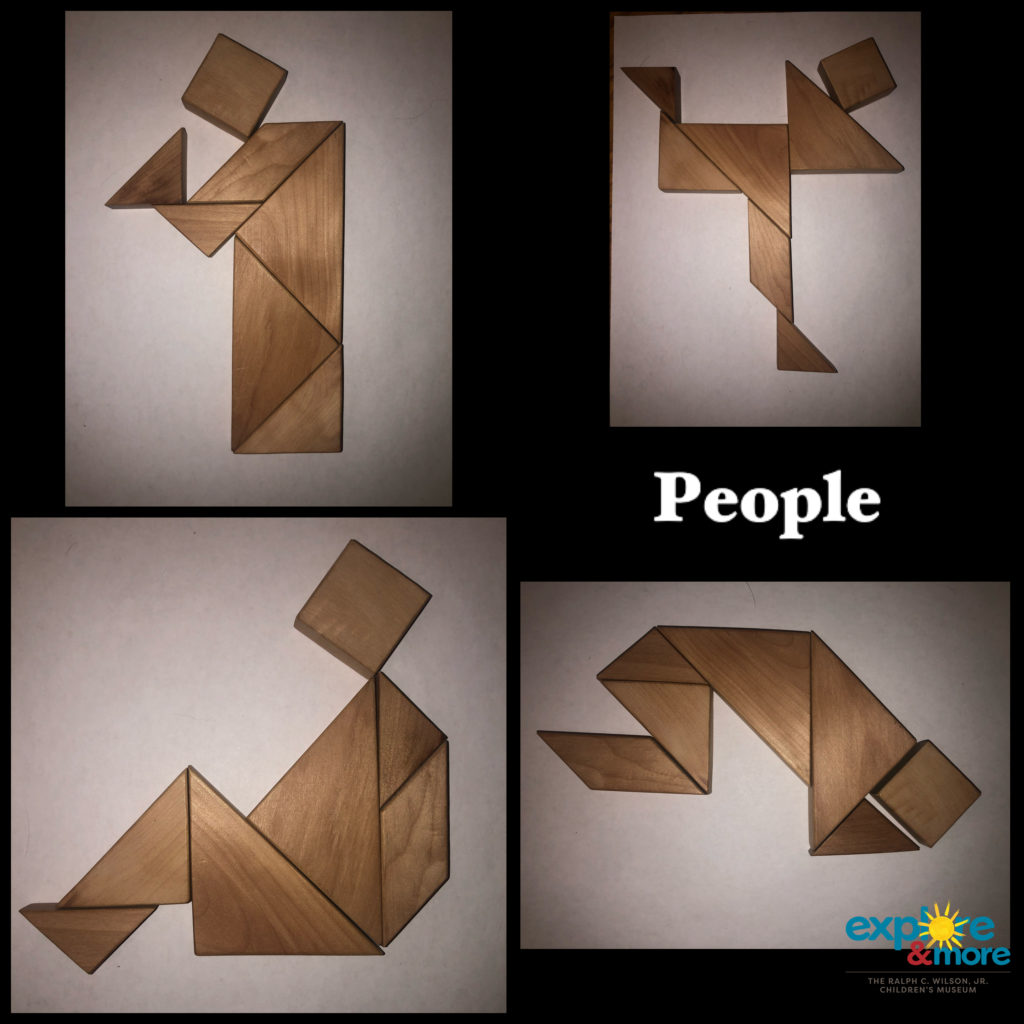
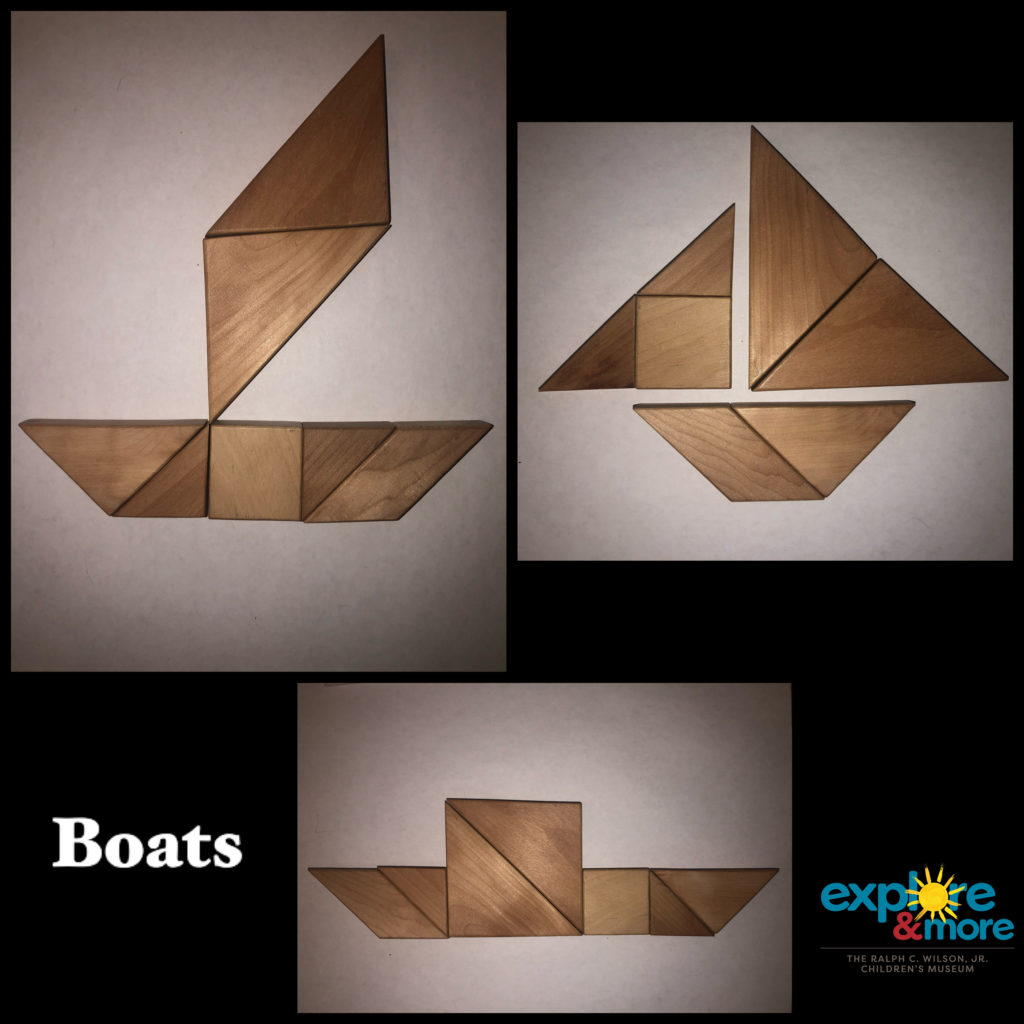
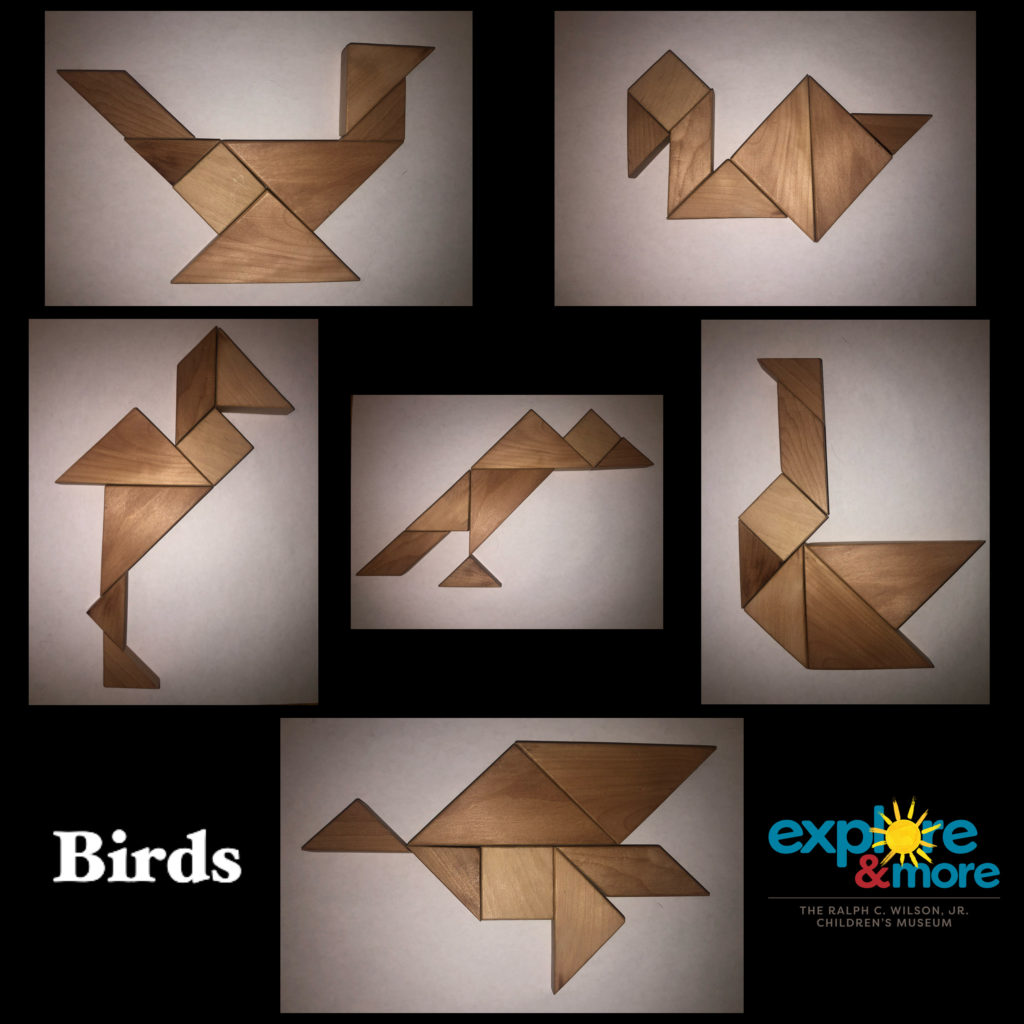
Step 4: Try to recreate the different figures illustrated below. Remember, you must use all seven of the tangram shapes, and they cannot overlap! We used our puzzle pieces to make a cat.
Hint: The figures that reveal the different shapes and colors are easier to solve. The silhouetted figures are much more difficult! You can find many more tangram challenges online and in books. Challenge yourself!
·It can be just as hard to arrange all of the tangram pieces back into a square – give it a try! How many different configurations can you find?
· How many different squares can you make with the smaller shapes? How many rectangles? How many triangles? Can you build a triangle with only two of the shapes? How about with three, four, five, or six?
Fun Facts:
· Tangram paradoxes or “dissection fallacies” occur when two figures are made using the same number of shapes, but one appears to be a subset of the other or to have pieces missing. One example is the “Magic Vases,” shown below, where some of the vase figures seem to have shapes missing, even though they are all made from the same seven tangram shapes.
·The children’s book, Grandfather Tang’s Story, by Ann Tompert, incorporates tangrams directly into the story and provides some good puzzles to try as you read.